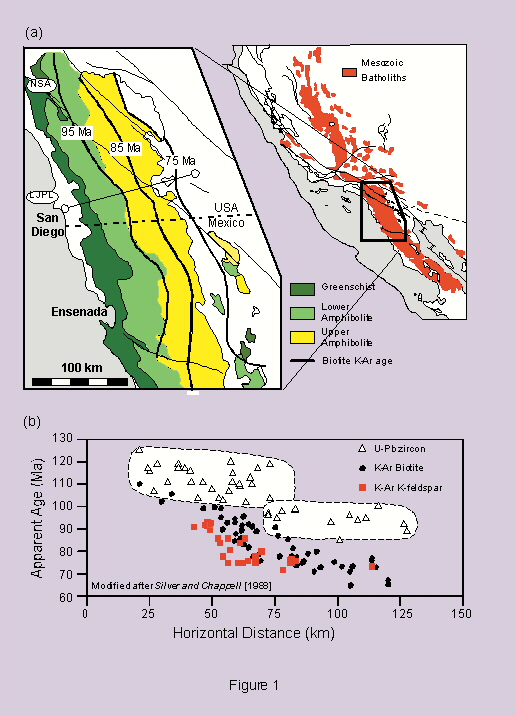
 Fig.1:
(a) Location of northern Peninsular Ranges batholith (PRB). Inset shows
distribution of batholith rocks, pattern of cooling ages [modified after
Krummenacher et al., 1975], and erosion depth [modified after Gastil, 1979]
throughout southern and Baja California. NSA (northern Santa Ana
mountains) and LJPL (La Jolla/Point Loma) denote sample localities (b)
Projection of available U-Pb zircon [Silver and Chappell, 1988; Walawender
et al., 1990; and Kimbrough, unpublished.] and K-Ar biotite and K-feldspar
ages [Krummenacher et al., 1975; Grove, 1994, Grove,unpublished] into X-X'
in (a). Fig.1:
(a) Location of northern Peninsular Ranges batholith (PRB). Inset shows
distribution of batholith rocks, pattern of cooling ages [modified after
Krummenacher et al., 1975], and erosion depth [modified after Gastil, 1979]
throughout southern and Baja California. NSA (northern Santa Ana
mountains) and LJPL (La Jolla/Point Loma) denote sample localities (b)
Projection of available U-Pb zircon [Silver and Chappell, 1988; Walawender
et al., 1990; and Kimbrough, unpublished.] and K-Ar biotite and K-feldspar
ages [Krummenacher et al., 1975; Grove, 1994, Grove,unpublished] into X-X'
in (a). |
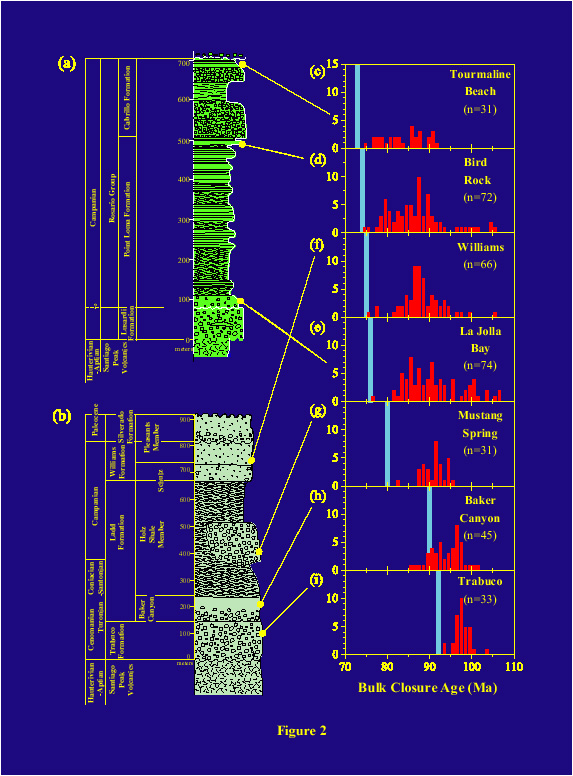 Fig.2: Stratigraphy of PRB forearc strata
in (a) San Diego area and (b) northern Santa Ana Mountains. (c)-(i)
Histograms of measured detrital K-feldspar closure age distributions.
Fig.2: Stratigraphy of PRB forearc strata
in (a) San Diego area and (b) northern Santa Ana Mountains. (c)-(i)
Histograms of measured detrital K-feldspar closure age distributions. |
 Fig.
3: Schematic of numerical model illustrating scaling and boundary conditions.
Light gray shading indicates portion of grid where closure ages are calculated.
Samples assigned to a given depositional age (AgeD) are from
±0.5 m.y. region. Bold line represents the final (65 Ma) erosion
surface. Fig.
3: Schematic of numerical model illustrating scaling and boundary conditions.
Light gray shading indicates portion of grid where closure ages are calculated.
Samples assigned to a given depositional age (AgeD) are from
±0.5 m.y. region. Bold line represents the final (65 Ma) erosion
surface. |
 Fig. 4: Progressive thermal development of
the batholith in Model II (Table 5). Net area intruded in each interval
indicated in lower left-hand panel corners. (a)-(f) Isothermal sections
prior to the onset of denudation (115, 113, 111, 109, 107, and 105 Ma);
(h-k) Isothermal sections for times characterized by simultaneous intrusion
and denudation (103, 101, 99, 97, and 95 Ma). Active erosion surfaces represented
by bold lines; (l) isothermal distribution at 65 Ma.
Fig. 4: Progressive thermal development of
the batholith in Model II (Table 5). Net area intruded in each interval
indicated in lower left-hand panel corners. (a)-(f) Isothermal sections
prior to the onset of denudation (115, 113, 111, 109, 107, and 105 Ma);
(h-k) Isothermal sections for times characterized by simultaneous intrusion
and denudation (103, 101, 99, 97, and 95 Ma). Active erosion surfaces represented
by bold lines; (l) isothermal distribution at 65 Ma. |
 Fig.
5: Contours of emplacement age for final pluton distributions in (a)
I1and (b) I2 (Table
3). (c) Mean intrusion age of plutonic rocks vs. horizontal distance.
Values calculated for I1 and I2 represented by solid and dashed lines respectively.
Superposed symbols represent U-Pb zircon age determined for PRB plutons
(see Figure 1 legend). Fig.
5: Contours of emplacement age for final pluton distributions in (a)
I1and (b) I2 (Table
3). (c) Mean intrusion age of plutonic rocks vs. horizontal distance.
Values calculated for I1 and I2 represented by solid and dashed lines respectively.
Superposed symbols represent U-Pb zircon age determined for PRB plutons
(see Figure 1 legend). |
 Fig. 6: Kolmogorov-Smirnov (K-S) statistical comparison of model
and measured closure age distributions. (a) Variation of significance
level of K-S statistic (PROB) in comparing model and measured distributions
for Mustang Spring sample. Depositional age and uncertainty for Mustang
Spring sample indicated above. (b)-(f) Comparison of model (light
gray) and measured results (black) for AgeD = 87, 85,
83, 81, and 79 Ma. All data normalized to 100.
Fig. 6: Kolmogorov-Smirnov (K-S) statistical comparison of model
and measured closure age distributions. (a) Variation of significance
level of K-S statistic (PROB) in comparing model and measured distributions
for Mustang Spring sample. Depositional age and uncertainty for Mustang
Spring sample indicated above. (b)-(f) Comparison of model (light
gray) and measured results (black) for AgeD = 87, 85,
83, 81, and 79 Ma. All data normalized to 100. |
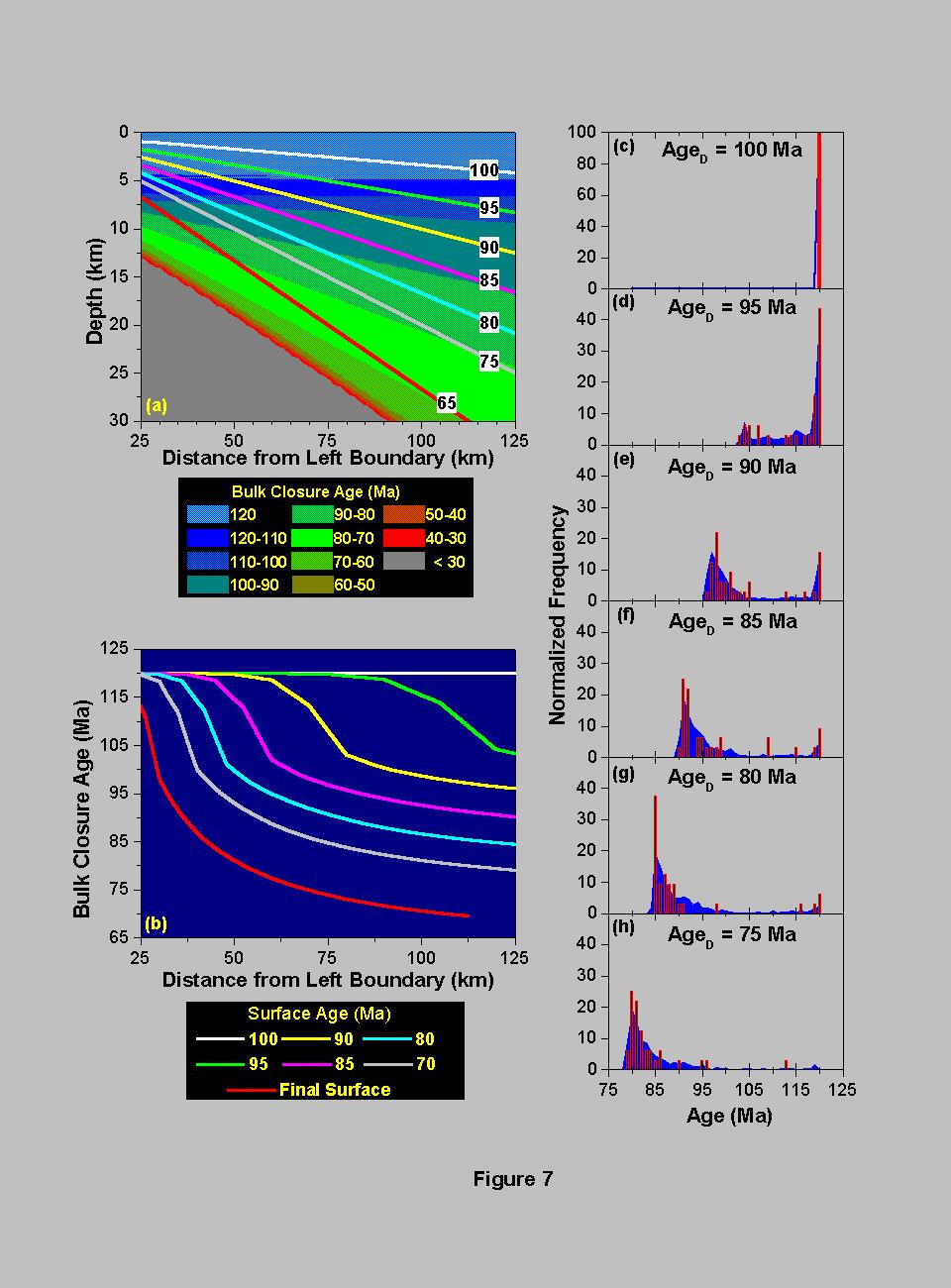 Fig.
7: Model I results: (a) Contour plot of final f K-feldspar bulk
closure age distribution (10 Ma contour interval). Surface positions
every 5 m.y. after initial denudation represented by labeled black lines.
Final (65 Ma) surface is bold line; (b) basement surface mineral
age profiles at indicated times; (c)-(h) Histograms of detrital K-feldspar
closure ages at indicated times. For each stratigraphic horizon,
we overlay distributions calculated from 32 (black) and 1000 (light gray)
random samples. All data normalized to 100. Fig.
7: Model I results: (a) Contour plot of final f K-feldspar bulk
closure age distribution (10 Ma contour interval). Surface positions
every 5 m.y. after initial denudation represented by labeled black lines.
Final (65 Ma) surface is bold line; (b) basement surface mineral
age profiles at indicated times; (c)-(h) Histograms of detrital K-feldspar
closure ages at indicated times. For each stratigraphic horizon,
we overlay distributions calculated from 32 (black) and 1000 (light gray)
random samples. All data normalized to 100. |
 Fig. 8: Model II results. Figure 7 caption contains explanation
of (a)-(h).
Fig. 8: Model II results. Figure 7 caption contains explanation
of (a)-(h). |
 Fig.
9: (a) Depositional ages and uncertainties for each samples (see
Table 1).
(b) -(g) Model I-VI results (see Figure 6 caption). PROBmax values
represented by symbols defined in (a) above (see Table 5). (h).
?t vs. depositional age models based upon (b)-(g) above. Fig.
9: (a) Depositional ages and uncertainties for each samples (see
Table 1).
(b) -(g) Model I-VI results (see Figure 6 caption). PROBmax values
represented by symbols defined in (a) above (see Table 5). (h).
?t vs. depositional age models based upon (b)-(g) above. |
 Fig. 10: Effect of radiogenic daughter product retentivity
upon Model II results (see Table
2). (a) apatite ((U-Th)-He); (b) K-feldspar, (c) biotite, (d)
hornblende (K-Ar); and (e) monazite (Th-Pb) age distributions. (f) Closure
ages variation along final (65 Ma) surface.
Fig. 10: Effect of radiogenic daughter product retentivity
upon Model II results (see Table
2). (a) apatite ((U-Th)-He); (b) K-feldspar, (c) biotite, (d)
hornblende (K-Ar); and (e) monazite (Th-Pb) age distributions. (f) Closure
ages variation along final (65 Ma) surface. |
 Fig.
11: Ability of variable rate denudation models to fit measured
detrital closure age distributions. (a) Depositional ages and uncertainties
for measured samples. (b)-(d) Model VII-IX results (see Figure 9 caption
for explanation; Table
5). (e) Deviation of AgeDmax from estimated stratigraphic ages
in models VII-IX. (f)-(l) Comparison of measured detrital closure
age with those of Model IX. All data normalized to 100. Fig.
11: Ability of variable rate denudation models to fit measured
detrital closure age distributions. (a) Depositional ages and uncertainties
for measured samples. (b)-(d) Model VII-IX results (see Figure 9 caption
for explanation; Table
5). (e) Deviation of AgeDmax from estimated stratigraphic ages
in models VII-IX. (f)-(l) Comparison of measured detrital closure
age with those of Model IX. All data normalized to 100. |
 Fig. 12: (a) Mean denudation rate vs. time for indicated models
(b) Histogram of bulk closure ages (= total fusion ages) calculated from
40Ar/39Ar step-heating of K-feldspars from east central PRB (see Figure
1 caption for data sources). (c)-(e) Comparison of predicted surface
ages from models I-IX with measured basement K-feldspar results vs. horizontal
distance.
Fig. 12: (a) Mean denudation rate vs. time for indicated models
(b) Histogram of bulk closure ages (= total fusion ages) calculated from
40Ar/39Ar step-heating of K-feldspars from east central PRB (see Figure
1 caption for data sources). (c)-(e) Comparison of predicted surface
ages from models I-IX with measured basement K-feldspar results vs. horizontal
distance. |
 Fig.2: Stratigraphy of PRB forearc strata
in (a) San Diego area and (b) northern Santa Ana Mountains. (c)-(i)
Histograms of measured detrital K-feldspar closure age distributions.
Fig.2: Stratigraphy of PRB forearc strata
in (a) San Diego area and (b) northern Santa Ana Mountains. (c)-(i)
Histograms of measured detrital K-feldspar closure age distributions. Fig.
3: Schematic of numerical model illustrating scaling and boundary conditions.
Light gray shading indicates portion of grid where closure ages are calculated.
Samples assigned to a given depositional age (AgeD) are from
±0.5 m.y. region. Bold line represents the final (65 Ma) erosion
surface.
Fig.
3: Schematic of numerical model illustrating scaling and boundary conditions.
Light gray shading indicates portion of grid where closure ages are calculated.
Samples assigned to a given depositional age (AgeD) are from
±0.5 m.y. region. Bold line represents the final (65 Ma) erosion
surface. Fig. 4: Progressive thermal development of
the batholith in Model II (Table 5). Net area intruded in each interval
indicated in lower left-hand panel corners. (a)-(f) Isothermal sections
prior to the onset of denudation (115, 113, 111, 109, 107, and 105 Ma);
(h-k) Isothermal sections for times characterized by simultaneous intrusion
and denudation (103, 101, 99, 97, and 95 Ma). Active erosion surfaces represented
by bold lines; (l) isothermal distribution at 65 Ma.
Fig. 4: Progressive thermal development of
the batholith in Model II (Table 5). Net area intruded in each interval
indicated in lower left-hand panel corners. (a)-(f) Isothermal sections
prior to the onset of denudation (115, 113, 111, 109, 107, and 105 Ma);
(h-k) Isothermal sections for times characterized by simultaneous intrusion
and denudation (103, 101, 99, 97, and 95 Ma). Active erosion surfaces represented
by bold lines; (l) isothermal distribution at 65 Ma. Fig.
5: Contours of emplacement age for final pluton distributions in (a)
I1and (b) I2 (Table
3). (c) Mean intrusion age of plutonic rocks vs. horizontal distance.
Values calculated for I1 and I2 represented by solid and dashed lines respectively.
Superposed symbols represent U-Pb zircon age determined for PRB plutons
(see Figure 1 legend).
Fig.
5: Contours of emplacement age for final pluton distributions in (a)
I1and (b) I2 (Table
3). (c) Mean intrusion age of plutonic rocks vs. horizontal distance.
Values calculated for I1 and I2 represented by solid and dashed lines respectively.
Superposed symbols represent U-Pb zircon age determined for PRB plutons
(see Figure 1 legend). Fig.
7: Model I results: (a) Contour plot of final f K-feldspar bulk
closure age distribution (10 Ma contour interval). Surface positions
every 5 m.y. after initial denudation represented by labeled black lines.
Final (65 Ma) surface is bold line; (b) basement surface mineral
age profiles at indicated times; (c)-(h) Histograms of detrital K-feldspar
closure ages at indicated times. For each stratigraphic horizon,
we overlay distributions calculated from 32 (black) and 1000 (light gray)
random samples. All data normalized to 100.
Fig.
7: Model I results: (a) Contour plot of final f K-feldspar bulk
closure age distribution (10 Ma contour interval). Surface positions
every 5 m.y. after initial denudation represented by labeled black lines.
Final (65 Ma) surface is bold line; (b) basement surface mineral
age profiles at indicated times; (c)-(h) Histograms of detrital K-feldspar
closure ages at indicated times. For each stratigraphic horizon,
we overlay distributions calculated from 32 (black) and 1000 (light gray)
random samples. All data normalized to 100. Fig. 8: Model II results. Figure 7 caption contains explanation
of (a)-(h).
Fig. 8: Model II results. Figure 7 caption contains explanation
of (a)-(h). Fig.
9: (a) Depositional ages and uncertainties for each samples (see
Table 1).
(b) -(g) Model I-VI results (see Figure 6 caption). PROBmax values
represented by symbols defined in (a) above (see Table 5). (h).
?t vs. depositional age models based upon (b)-(g) above.
Fig.
9: (a) Depositional ages and uncertainties for each samples (see
Table 1).
(b) -(g) Model I-VI results (see Figure 6 caption). PROBmax values
represented by symbols defined in (a) above (see Table 5). (h).
?t vs. depositional age models based upon (b)-(g) above. Fig. 10: Effect of radiogenic daughter product retentivity
upon Model II results (see Table
2). (a) apatite ((U-Th)-He); (b) K-feldspar, (c) biotite, (d)
hornblende (K-Ar); and (e) monazite (Th-Pb) age distributions. (f) Closure
ages variation along final (65 Ma) surface.
Fig. 10: Effect of radiogenic daughter product retentivity
upon Model II results (see Table
2). (a) apatite ((U-Th)-He); (b) K-feldspar, (c) biotite, (d)
hornblende (K-Ar); and (e) monazite (Th-Pb) age distributions. (f) Closure
ages variation along final (65 Ma) surface. Fig.
11: Ability of variable rate denudation models to fit measured
detrital closure age distributions. (a) Depositional ages and uncertainties
for measured samples. (b)-(d) Model VII-IX results (see Figure 9 caption
for explanation; Table
5). (e) Deviation of AgeDmax from estimated stratigraphic ages
in models VII-IX. (f)-(l) Comparison of measured detrital closure
age with those of Model IX. All data normalized to 100.
Fig.
11: Ability of variable rate denudation models to fit measured
detrital closure age distributions. (a) Depositional ages and uncertainties
for measured samples. (b)-(d) Model VII-IX results (see Figure 9 caption
for explanation; Table
5). (e) Deviation of AgeDmax from estimated stratigraphic ages
in models VII-IX. (f)-(l) Comparison of measured detrital closure
age with those of Model IX. All data normalized to 100. Fig. 12: (a) Mean denudation rate vs. time for indicated models
(b) Histogram of bulk closure ages (= total fusion ages) calculated from
40Ar/39Ar step-heating of K-feldspars from east central PRB (see Figure
1 caption for data sources). (c)-(e) Comparison of predicted surface
ages from models I-IX with measured basement K-feldspar results vs. horizontal
distance.
Fig. 12: (a) Mean denudation rate vs. time for indicated models
(b) Histogram of bulk closure ages (= total fusion ages) calculated from
40Ar/39Ar step-heating of K-feldspars from east central PRB (see Figure
1 caption for data sources). (c)-(e) Comparison of predicted surface
ages from models I-IX with measured basement K-feldspar results vs. horizontal
distance.